In four dimensions, the Minkowski metric  leads to the 16-dimensional Clifford algebra C(1,3), Dirac's equation [1] is using four of these 16 matrices that form a basis of this algebra, a new operator is defined using all of these matrices and also generalized for a curved space. This new multilevel operator generalizes the Dirac's equation, the value of the generalized Dirac's operator is calculated in the Schwarzschild's metric. The torsion tensor is calculated taking into account the non-symmetric part of the metric tensor in the vanishing of its covariant derivative and applied to Kerr's metric generalizing the Clifford algebra. Geodesic equation, conservation laws, torsion tensor and Einstein field equation are obtained in a non-symmetric geometry.
leads to the 16-dimensional Clifford algebra C(1,3), Dirac's equation [1] is using four of these 16 matrices that form a basis of this algebra, a new operator is defined using all of these matrices and also generalized for a curved space. This new multilevel operator generalizes the Dirac's equation, the value of the generalized Dirac's operator is calculated in the Schwarzschild's metric. The torsion tensor is calculated taking into account the non-symmetric part of the metric tensor in the vanishing of its covariant derivative and applied to Kerr's metric generalizing the Clifford algebra. Geodesic equation, conservation laws, torsion tensor and Einstein field equation are obtained in a non-symmetric geometry.
Gravitomagnetic Tensor; Gravitational Magnetic Field; Energymomentum1-Form; Clifford Algebra; Dirac Equation; Dirac Operator; Gravity and Quantum Mechanics Unification; Multilevel Operator; Schwarzschild's Metric; Torsion Tensor; Rearranged Kerr's Metric; Generalized Clifford Algebra; Generalized Einstein Field Equation; Generalized Geodesic Equation; Conservation Laws; Non-Symmetric Geometry
Dirac's equation is the relativistic wave equation derived by physicist Paul Dirac in 1928. The wave functions in the Dirac theory are vectors of four complex components (known as bispinors), two of which resemble the Pauli wavefunction in the non-relativistic limit, in contrast to the Schrodinger equation which described wave functions of only one complex component.
Dirac's operator is just the tip of the iceberg, the tip of a generalized operator that is obtained by operating on all members of the Clifford algebra basis and not just on four of them.
The Schwarzschild's metric is named in honour of Karl Schwarzschild, who found the exact solution in 1915 and published it in January 1916, a little more than a month after the publication of Einstein's theory of general relativity. It was the first exact solution of the Einstein field equations other than the trivial at space solution. Schwarzschild died shortly after his paper was published, as a result of a disease he developed while serving in the German army during World War I. Johannes Droste in 1916 independently produced the same solution as Schwarzschild.
Schwarzschild's metric is an exact solution to the Einstein's field equations that describes the gravitational field outside a spherical mass, on the assumption that the electric charge of the mass, angular momentum of the mass, and universal cosmological constant is all zero.
The new generalized Dirac's operator, the multilevel operator, is calculated in the Schwarzschild's metric, torsion tensor and new gravitomagnetic tensor appear in level 2, curvature tensor appears in levels 3 and 4.
The Kerr's metric is a generalization to a rotating body of the Schwarzschild's metric. The Einstein field equation relates the geometry of spacetime to the distribution of matter within it. The equations were published by Einstein in 1915 in the form of a tensor equation which related the local spacetime curvature with the local energy, momentum and stress within that spacetime expressed by the stress-energy tensor.
Multilevel operator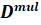
We are using Pauli matrices σ, electromagnetic four-potential 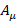 and charge e with
and charge e with 
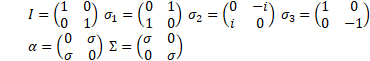 1
1
In four dimensions, Minkowski's metric ημν = diag (+1,-1,-1,-1) leads to the Clifford algebra C(1,3)[2], 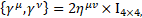 Dirac matrices
Dirac matrices 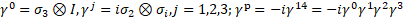
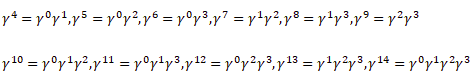 2
2
Multilevel operator Dn acts on level n, n is the number of matrices in the product of the algebra members, for example, D3 acts on γ10, γ11, γ12, and γ13. Total multilevel operator 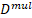 = D0 +D1 +D2 +D3 +D4, the action of
= D0 +D1 +D2 +D3 +D4, the action of 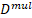 on the spinor function vanishes
on the spinor function vanishes 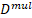 ψ=0 3
ψ=0 3
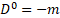 4
4
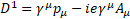 5
5
 6
6
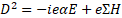 7
7
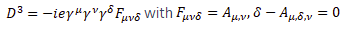 8
8
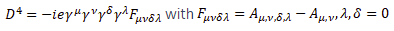 9
9
Multilevel operator 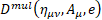 can be generalized for a curved space with four-potential P, field charge q and covariant derivative [3]
can be generalized for a curved space with four-potential P, field charge q and covariant derivative [3] 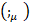 instead of derivative
instead of derivative  in the definition of
in the definition of 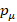
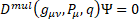 10
10
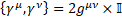 11
11
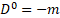 12
12
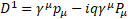 13
13
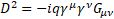 with
with 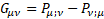 14
14
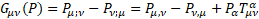 15
15
 16
16
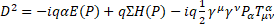 17
17
For gravity 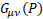 is the new gravitomagnetic tensor.
is the new gravitomagnetic tensor. 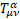 is the torsion tensor [4].
is the torsion tensor [4].
 18
18
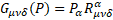 with
with 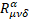 the Riemann-Christoffel tensor [5]. 19
the Riemann-Christoffel tensor [5]. 19
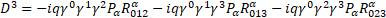
 20
20
 21
21
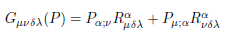 22
22
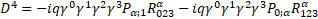 23
23
We are using 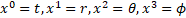 with
with  , this metric is defined by [6]
, this metric is defined by [6]
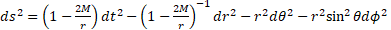 24
24
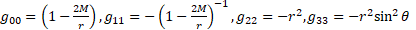 25
25
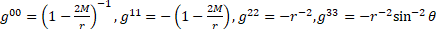 26
26
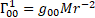 27
27
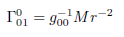 28
28
 29
29
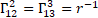 30
30
 31
31
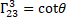 32
32
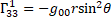 33
33
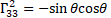 34
34
 35
35
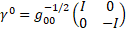 36
36
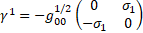 37
37
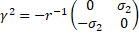 38
38
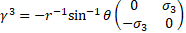 39
39
 , from equations (16) and (17) 40
, from equations (16) and (17) 40
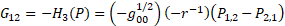 41
41
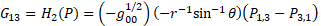 42
42
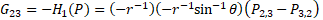 43
43
 44
44
 45
45
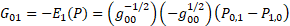 46
46
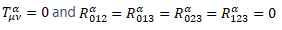 47
47
Energy-momentum form is a 1-form [7]
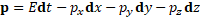 48
48
dp is a 2-form
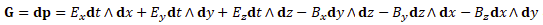 49
49
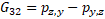 50
50
 51
51
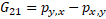 52
52
Comparing equations (41-43) and (50-52) we can infer
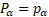 53
53
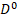 is related to the scalar 0 -form
is related to the scalar 0 -form  is related to the Energy-momentum 1-form,
is related to the Energy-momentum 1-form, 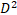 is related to the Electromagnetic 2 -form,
is related to the Electromagnetic 2 -form, 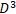 is related to
is related to
 3-form [8].
3-form [8].
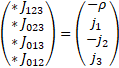 54
54
 is related to L 4-form [9].
is related to L 4-form [9].
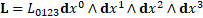 55
55
 is the proyector matrix, historically
is the proyector matrix, historically 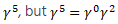
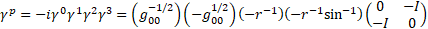 56
56
We are using 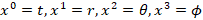 M is the black hole's mass and a is the angular momentum per unit mass with G = c = 1. The invariance of the length of vectors under parallel transport means that the connection is compatible with the metric, it is a metric connection, the requirement of the preservation of the length by parallel transport may be stated as [10].
M is the black hole's mass and a is the angular momentum per unit mass with G = c = 1. The invariance of the length of vectors under parallel transport means that the connection is compatible with the metric, it is a metric connection, the requirement of the preservation of the length by parallel transport may be stated as [10].
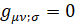 57
57
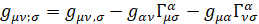 58
58
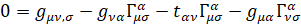 , with
, with 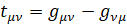 59
59
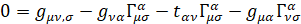 60
60
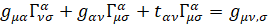 61
61
 62
62
Solving these equations, we get the torsion applying its definition [11].
 63
63
Expanding the line element in powers of 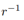 and examining the leading terms [12].
and examining the leading terms [12].

 64
64
Rearranging the line elements
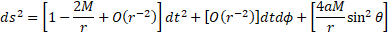
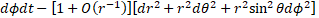 65
65
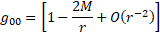 66
66
 67
67
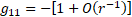 68
68
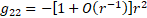 69
69
 70
70
 71
71
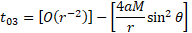 72
72
 73
73
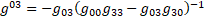 74
74
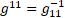 75
75
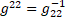 76
76
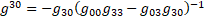 77
77
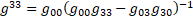 78
78
Generalizing Clifford algebra with 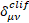 , if
, if 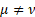 and
and 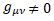 then 1, else 0
then 1, else 0
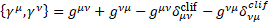 79
79
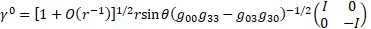 80
80
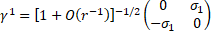 81
81
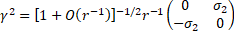 82
82
 83
83
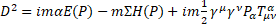 , from equations (16) and (17) 84
, from equations (16) and (17) 84
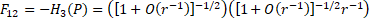
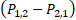 85
85
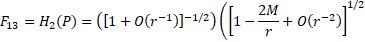
 86
86

 87
87
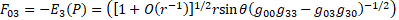
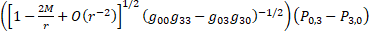 88
88
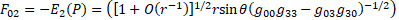
 89
89
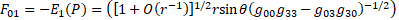
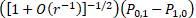 90
90
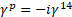 is the proyector matrix, historically
is the proyector matrix, historically 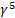 , but
, but 

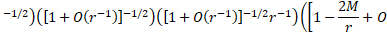
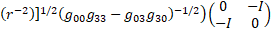 91
91
A geodesic that is not a null geodesic has the property that 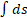 , taken along a section of the track with the end points
, taken along a section of the track with the end points 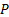 and
and 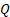 , is stationary if one makes a small variation of the track keeping the end points fixed. If
, is stationary if one makes a small variation of the track keeping the end points fixed. If 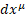 denotes an element along the track [13].
denotes an element along the track [13].
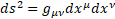 92
92
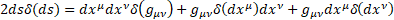 93
93
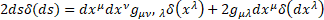 94
94
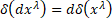 and
and 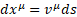 95
95
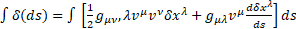 96
96
By partial integration with 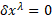 at end points
at end points 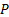 and Q, we get
and Q, we get
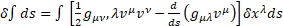 97
97
The condition for this to vanish with arbitrary  is
is
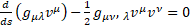 98
98
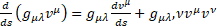 99
99
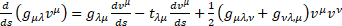 , and with
, and with  100
100
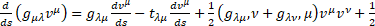
 101
101
From equation (98) with 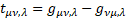
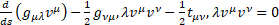 102
102
Thus the condition (102) becomes
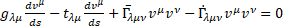 103
103
 104
104
 105
105
Multiplying equation (103) by 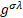 , we obtain the geodesic equation
, we obtain the geodesic equation
 106
106
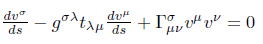 107
107
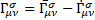 108
108
 are the Christoffel symbols of the symmetric part, so
are the Christoffel symbols of the symmetric part, so
 109
109
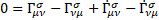 110
110
We directly obtain the torsion tensor without solving equations (62) and (63)
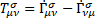 111
111
From equation (108) where  are the symbols of the symmetric part
are the symbols of the symmetric part
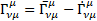 112
112
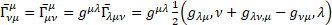 113
113
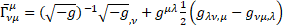 114
114
Equation (112) becomes
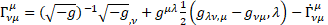 115
115
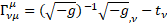 116
116
 117
117
The vector 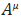 has the covariant divergence
has the covariant divergence
 118
118
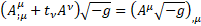 119
119
If the left-hand side of equation (119) equals zero then the right-hand side gives us the first conservation law.
For the antisymmetric tensor 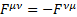
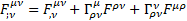 120
120
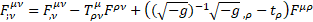 121
121
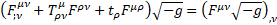 122
122
If the left-hand side of equation (122) equals zero then the right-hand side gives us the second conservation law.
For the antisymmetric tensor 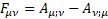
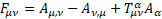 123
123
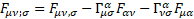 124
124
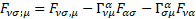 125
125
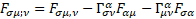 126
126
Adding equations (124), (125) and (126)
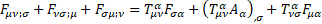
 127
127
From the definition of the curvature tensor 
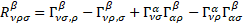 128
128
 is called the Ricci tensor
is called the Ricci tensor
 129
129
Now 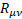 is not symmetric,
is not symmetric, 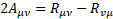 is the antisymmetric part and
is the antisymmetric part and 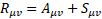 where
where 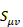 is the symmetric part in the Einstein's equation [14].
is the symmetric part in the Einstein's equation [14].
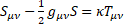 130
130
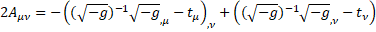 ,
,
 131
131
 132
132
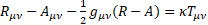 133
133
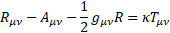 134
134
Multilevel operator 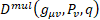 has been generalized for a curved space with a general four-potential P. For gravity
has been generalized for a curved space with a general four-potential P. For gravity  is the new gravitomagnetic tensor and torsion tensor
is the new gravitomagnetic tensor and torsion tensor  appears in its definition.
appears in its definition.
In a at space 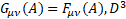 and
and  operators vanish. In a curved space the curvature tensor
operators vanish. In a curved space the curvature tensor 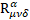 appears in levels 3 and 4.
appears in levels 3 and 4.
The appearance of torsion tensor  and curvature tensor
and curvature tensor 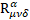 in multilevel operator
in multilevel operator 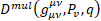 means that this operator is a fundamental operator in Quantum Field Theory.
means that this operator is a fundamental operator in Quantum Field Theory.
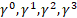 , have been calculated for Schwarzschild's metric, then
, have been calculated for Schwarzschild's metric, then  , the gravitomagnetic tensor has been obtained.
, the gravitomagnetic tensor has been obtained.
Each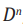 , where n is the number of γ matrices in the product of the algebra members, is related to an n-form.
, where n is the number of γ matrices in the product of the algebra members, is related to an n-form.
The invariance of the length of vectors under parallel transport requires the vanishing of the metric tensor covariant derivative, a new term appears in equation (59) with 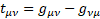 measuring the non-symmetric part of the metric tensor, solving these equations we get the torsion tensor.
measuring the non-symmetric part of the metric tensor, solving these equations we get the torsion tensor.
Rearranging Kerr's metric we obtained 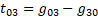 , the non-symmetric part of the metric tensor, gravitomagnetic tensor has also been calculated generalizing the Clifford algebra.
, the non-symmetric part of the metric tensor, gravitomagnetic tensor has also been calculated generalizing the Clifford algebra.
Taking into account the 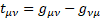 in the geodesic equation we have obtained the torsion tensor, conservation laws and Einstein field equation in a non-symmetric geometry.
in the geodesic equation we have obtained the torsion tensor, conservation laws and Einstein field equation in a non-symmetric geometry.
- Marian Fecko (2006) Differential Geometry and Lie Groups for Physicists. Cambridge University Press 637.
- Marian Fecko (2006) Differential Geometry and Lie Groups for Physicists. Cambridge University Press 650652.
- Marian Fecko (2006) Differential Geometry and Lie Groups for Physicists. Cambridge University Press 380.
- Marian Fecko (2006) Differential Geometry and Lie Groups for Physicists. Cambridge University Press 384.
- Marian Fecko (2006) Differential Geometry and Lie Groups for Physicists. Cambridge University Press 404.
- P.A.M. Dirac (1996) General Theory of Relativity. Princeton University Press 30-32.
- J.A. Wheeler., C. Misner., K.S. Thorne (2017) Gravitation. Princeton University Press 91.
- Roger Penrose (2006) El camino a la realidad. Random House Mondadori, Barcelona 603.
- J.A. Wheeler., C. Misner., K.S. Thorne (2017) Gravitation. Princeton University Press 119.
- Marian Fecko (2006) Differential Geometry and Lie Groups for Physicists. Cambridge University Press 383.
- Marian Fecko (2006) Differential Geometry and Lie Groups for Physicists. Cambridge University Press 389.
- J.A. Wheeler., C. Misner., K.S. Thorne (2017) Gravitation. Princeton University Press 891.
- P.A.M. Dirac (1996) General Theory of Relativity. Princeton University Press 16-17.
- J.A. Wheeler., C. Misner., K.S. Thorne (2017) Gravitation. Princeton University Press 406.

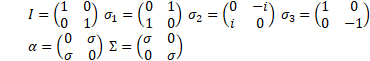 1
1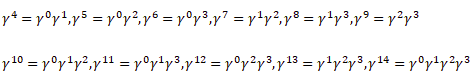 2
2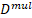 = D0 +D1 +D2 +D3 +D4, the action of
= D0 +D1 +D2 +D3 +D4, the action of  6
6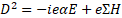 7
7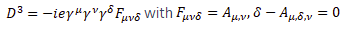 8
8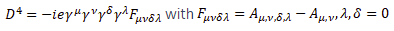 9
9 instead of derivative
instead of derivative  in the definition of
in the definition of 
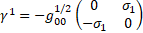 37
37 , from equations (16) and (17) 40
, from equations (16) and (17) 40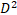 is related to the Electromagnetic 2 -form,
is related to the Electromagnetic 2 -form, 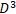 is related to
is related to 3-form [8].
3-form [8].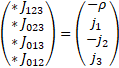 54
54 is related to L 4-form [9].
is related to L 4-form [9]. 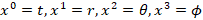 M is the black hole's mass and a is the angular momentum per unit mass with G = c = 1. The invariance of the length of vectors under parallel transport means that the connection is compatible with the metric, it is a metric connection, the requirement of the preservation of the length by parallel transport may be stated as [10].
M is the black hole's mass and a is the angular momentum per unit mass with G = c = 1. The invariance of the length of vectors under parallel transport means that the connection is compatible with the metric, it is a metric connection, the requirement of the preservation of the length by parallel transport may be stated as [10].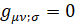 57
57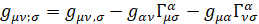 58
58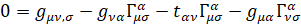 , with
, with 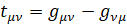 59
59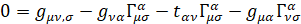 60
60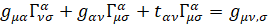 61
61 62
62 63
63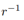 and examining the leading terms [12].
and examining the leading terms [12].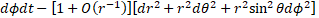 65
65 then 1, else 0
then 1, else 0 , taken along a section of the track with the end points
, taken along a section of the track with the end points  , is stationary if one makes a small variation of the track keeping the end points fixed. If
, is stationary if one makes a small variation of the track keeping the end points fixed. If 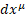 denotes an element along the track [13].
denotes an element along the track [13].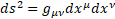 92
92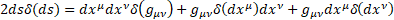 93
93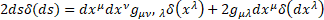 94
94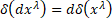 and
and 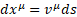 95
95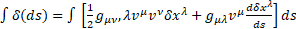 96
96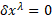 at end points
at end points 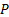 and Q, we get
and Q, we get 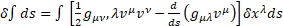 97
97 is
is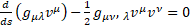 98
98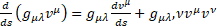 99
99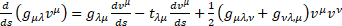 , and with
, and with  100
100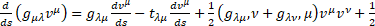
 101
101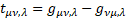
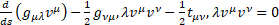 102
102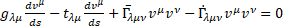 103
103 104
104 105
105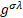 , we obtain the geodesic equation
, we obtain the geodesic equation 106
106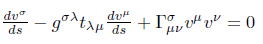 107
107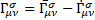 108
108 are the Christoffel symbols of the symmetric part, so
are the Christoffel symbols of the symmetric part, so 109
109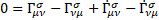 110
110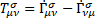 111
111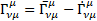 112
112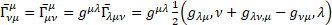 113
113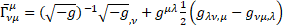 114
114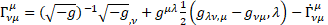 115
115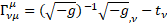 116
116 117
117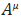 has the covariant divergence
has the covariant divergence 118
118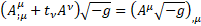 119
119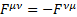
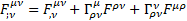 120
120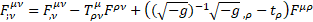 121
121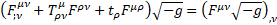 122
122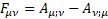
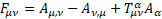 123
123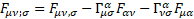 124
124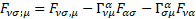 125
125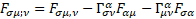 126
126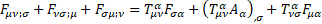
 127
127
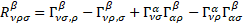 128
128 is called the Ricci tensor
is called the Ricci tensor 129
129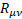 is not symmetric,
is not symmetric, 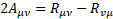 is the antisymmetric part and
is the antisymmetric part and 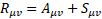 where
where 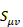 is the symmetric part in the Einstein's equation [14].
is the symmetric part in the Einstein's equation [14].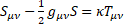 130
130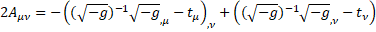 ,
, 131
131 132
132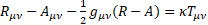 133
133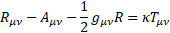 134
134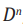 , where n is the number of γ matrices in the product of the algebra members, is related to an n-form.
, where n is the number of γ matrices in the product of the algebra members, is related to an n-form.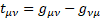 in the geodesic equation we have obtained the torsion tensor, conservation laws and Einstein field equation in a non-symmetric geometry.
in the geodesic equation we have obtained the torsion tensor, conservation laws and Einstein field equation in a non-symmetric geometry.