Abstract

In the Eigen Theory of the physical world, the fundamental form of a particle of matter is a distribution of points that forms a manifold of its own. Each point results from the intersection of a corresponding pair of eigenvelocity vectors, which are functions of a pair of symmetric and antisymmetric tensors characteristic of gravitation and electromagnetism respectively. The theory develops in three stages. The first and second stages produce the wave and particle aspects matter. The third stage formulates the base manifold, characterised by an eigenfield of a second pair of symmetric and antisymmetric tensors, in which the particle moves as a whole whilst rotating about an axis through the particle centre. The first two stages expose the reality behind matter-antimatter, the quantum mechanical wave-particle duality and the results of the double-slit experiment. The third stage reveals the nature of dark energy and the structure of dark matter. In Eigen Theory Quantum Mechanics becomes restructured; in particular the uncertainty principle ceases to exist.

Keywords

Pair of Eigenvelocity Vectors; Gravitation and Electromagnetism; Matter-Antimatter; Double-Slit Experiment; Dark Matter and Dark Energy; CMB Radiation; Fibre Bundle 

Introduction

The Eigen Theory (ET) this paper presents establishes that the fundamental form of a particle of matter, or simply the particle, is the key to the complete structure of the physical world.  The particle is a distribution of points that forms a manifold of its own. Each point results from the intersection of a corresponding pair of eigenvelocity vectors 𝒗 and 𝒖 that represents the wave aspect of the particle. The vectors 𝒗 and 𝒖 are functions of a pseudo-Riemannian symmetric metric tensor g and an antisymmetric tensor h. These two tensors are characteristics respectively of:

  (a) The magnitude of the vectors 𝒗 and 𝒖 and their angular separation

  (b) The concepts of mass and charge

  (c) Gravitation and electromagnetism

In Minkowski spacetime, 𝒗 and 𝒖 turn out to be Lorentzian boosts of each other. Thus, ET subsumes Special Relativity (SR) at the outset and it subsumes General Relativity (GR) in due course. ET has three primary sets of equations F_1, F₂ and F_3. These happen to be the respective ET counterparts of the three laws of Newton (§4.4). Therefore, in ET, theoretical physics may have completed a full circle and stands liberated high above the place on which it stood restricted at first.

The above outline of ET suggests that for ET, as it was for SR, no relevant fundamental references are available to cite. ET begins so to speak with a clean slate and finds that pairing is the primary feature of the physical world and pairs such as (𝒗, 𝒖), (g,h) and more besides are the vocabulary of the physical world. .

 In this paper, ET develops in 3-stages in §1, 2 and 3, as follows. The first stage in §1 produces the following.

(a) The vectors v and u as functions of g and h.

(b) The mutual transport equations of v and u resulting from an arbitrary infinitesimal perturbation of the point of inter- section of v and u that preserves their angular separation

(c) A set of field equations satisfied by g and h, which is a transformation of the above mutual transport equations

(d) Wave aspects of both the particle and the pair of fields g and h

In the second stage in §2 a simple transformation of 𝒗 and 𝒖 produces the particle. It is made up of the three fundamental pairs of opposites of the physical world, time-space, translation-rotation, and gravitation-electromagnetism. The two components of each of these pairs unify at the particle centre and this triple unification physically manifests as a pair of null photon velocity vectors. Thus, the particle centre is of an extraordinary character and is able to function as a fulcrum on which each of the three pairs of opposites maintains a perpetual state of dynamic balance.

The third stage in §3 formulates the base manifold in which the particle translates as a whole whilst also rotating about an axis through the particle centre. The characteristic feature of the base manifold is an Eigenfield of a second pair of symmetric and antisymmetric tensor fields g and h  In ET this pair of fields is the structure of the so-called dark matter.

The particle consists of two alternative forms that corresponds to the + and – signs of the antisymmetric tensor h (§2.1). How- ever, these may co-exist in a base manifold as two particles each with its own manifold one of which is charged positive and the other charged negative; they are the progenitors of hadron and lepton of particle physics. The fundamental form of this hadron-lepton pair is the Hydrogen atom. The internal and external structures of this atom are approximately electromagnetic and gravitational, respectively. Hence, these two structures are characterised by an approximate separation of electromagnetism and gravitation.  Since electromagnetic and gravitational motions are rotational and translational, these motions also separate. So also do space and time, as they are the spacetime subdivisions that correspond to rotation and translation, respectively. Thus, the triple unification of the three pairs of opposites, which manifests as the particle centre, effectively separate for the Hydrogen atom. Their eventual state of dynamic balance is what is central to evolution of the internal and external structures of the Hydrogen atom as atomic and cosmic regions of the physical world, respectively.

From the foregoing, it follows that the atomic and cosmic regions are a pair of opposites. Furthermore, in comparison to the cosmic region the atomic region is tiny. However, the electromagnetic force in this tiny region is mighty and the gravitational force in the massive cosmic region is feeble on average.

Just as the three fundamental pairs of opposites of the particle are dynamically balanced on the fulcrum, which is the particle centre, each of those three pairs of opposites, characteristic of the atomic and cosmic regions respectively, may also be dynamically balanced on a fulcrum of ‘immense complexity’, which is characteristic of the central region, or the macroscopic region, of the physical world where life evolves. An analysis of this balancing and the nature of the corresponding fulcrum are outside the scope of this paper as life itself may be the fundamental element of these.

According to the forgoing, a pair of fulcrums may exist, one physical and the other perhaps not.

§5 compute the motion of point-satellites of negligible masses that circle a static spherically symmetric cosmic central body. In §6 an atomic central body system is examined using the Hydrogen atom as a composite structure that represents static spherically symmetric fields.

1. Stage 1 of the Eigen Theory

1.1 The pair of eigenvelocity vectors 𝒗 and 𝒖

Appendix A develops the following set of algebraic equations F₁ satisfied by the vectors 𝒗 and 𝒖 and their eigenvalue β.

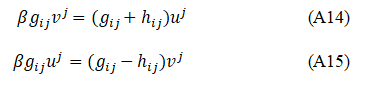
In n-spacetime F₁ produces n pairs of vectors 𝒗 and 𝒖 and n corresponding eigenvalues β as functions of g and h. The tensor h is the sum of a 2-form and the exterior derivative of a 1-form p, which means that h has the same number of elements as the tensor g. The elements of p are the counterparts of the elements of g that are arbitrary due to the Bianchi identities that g satisfies; hence, the elements of p are likewise primarily arbitrary. The tensors g and h, being the same for each of the n pairs of eigenvelocity vectors, are the kernel, or the substance, of matter. Therefore, in this introductory paper on ET it suffices to focus on just one pair of vectors, 𝒗 and 𝒖. Appendix A also shows that in Minkowski spacetime 𝒗 and 𝒖 are Lorentzian boosts of each other.

F₁ produces only squired values of β; hence β is positive or negative and these produce the following pairs of vectors.  

  (a) (±𝒗, ±𝒖) as a reversal of both 𝒗 and 𝒖 also satisfies F₁

  (b) (±𝒗, ∓𝒖) as according to F₁ if β changes its sign then either 𝒗 or 𝒖 reverses

Fig. 1 shows sketches of the pairs of vectors in (a) as (s1, s2) and those in (b)  as (s3, s4). In s1, the coordinate axes at the point of intersection of 𝒗 and 𝒖 are so configured that 𝒗 and 𝒖 are symmetrically placed with respect to the time-axis and co-planar with it.  This coordinate configuration may be considered as a form of MCRF.

According to F₁ if 𝒗 and 𝒖 are interchanged then h reverses its sign. In s1 and s2 this interchange reverses the spatial direction of each of 𝒗 and 𝒖. Accordingly F₁ has CP symmetry since the sign of h also corresponds to the sign of charge. In s3 and s4, if 𝒗 and 𝒖 are interchanged then time reverses for each of 𝒗 and 𝒖; accordingly, F₁ has CT symmetry. If both 𝒗 and 𝒖 are reversed then time reverses for each of these; accordingly F₁ has PT symmetry. Owing to the reversal of time, CT and PT symmetries are ‘hidden’. 

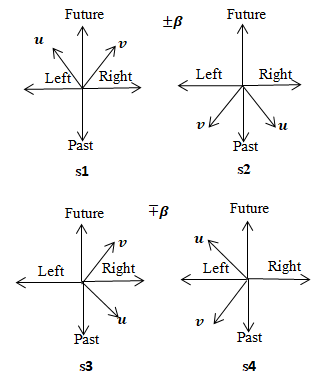
Figure 1. The two pairs of solutions of F₁

1.2 The absolute conservation principle

Multiplying (A14) by 𝒖 and (A15) by 𝒗 and combining the results we get

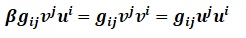(1)

These together with F₁ produce the following.

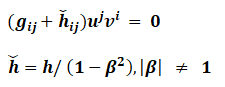(2) (2a)

The scalars and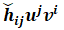in (2) are the ET counterparts of energy and angular momentum that are conserved separately in standard physics.  According to (2), in ET the sum of these two counterparts is conserved as zero in perpetuity.

Thus an absolute conservation principle of nothingness exists according to which the sum of energy and scalar angular momentum shared by the pair of eigenvelocity vectors remains zero in perpetuity.

By analogy with Euclidean geometry, (1) leads to

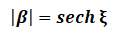(3)

where ξ is the hyperbolic angle between 𝒗 and 𝒖. Accordingly |β| is confined to the closed interval [0, 1]. Then (1) indicates that

(4)

Equation (2) can now be re-written as

(5)

Hence, in ET the fundamental parameters of energy and scalar angular momentum of 𝒗 and 𝒖 unify as β. Finally, 𝒗 and 𝒖 are:

(a) Of equal magnitudes, according to (1)

(b) Orthogonal with respect to the general tensor 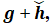according to (2)

(c) In a state of nothingness or nullity, according to (2)

(d) Such that according to F₁ if they unify then |β|=1 and h reduces to zero and F₁ becomes trivial. Hence, the vector of unification becomes indeterminate and in that sense, it becomes singular.

According to the above features, 𝒗 and 𝒖 possess Equality, Orthogonality, Nullity and Singularity, which bear the acronym EONS.

1.3 The CMB radiation

For the condition β= 0, (A14) and (A15) produce the following set of equations of the wave aspect of the particle. 

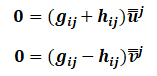(A19) (A20)

(A21)

The null velocities 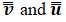may represent the CMB radiation. In that case, CMB radiation is integral with the state of relativity between the pair of vectors 𝒗 and 𝒖; hence, its presence is not in violation of the principle of relativity, as it appears to be at present, but is in conformity with it.

1.4 The transport equations of 𝒗 and 𝒖 and the field equation satisfied by g and h

In GR, the geodesic, which is a curve that parallel-transports its tangent vector, can be obtained using the criterion that an arbitrary infinitesimal perturbation of the geodesic does not alter its length between two fixed points.  In ET, the mutual transport equations of 𝒗 and 𝒖 are obtained using a similar procedure, which applies an arbitrary infinitesimal perturbation to the point of intersection of 𝒗 and 𝒖. In this case, the perturbation preserves β. In Appendix B, this procedure applied to (5) produces a pair of mutual transport equations satisfied by 𝒗 and 𝒖, given below as (B15) and (B16). In these 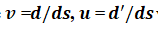where S is the path parameter.

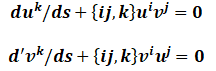		(B15) (B16)
where		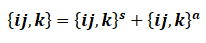(B17)

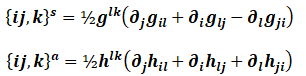(B4) (B9)

Equations (B15) and (B16) can be re-written as follows.

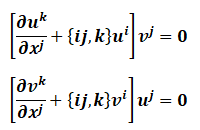(6) (7)

Since in n-spacetime g and h at present consists of n²+ n free variables, (6) can be reduced to

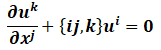(8)

or (7) can be reduced to

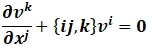(9)

Equations (8) and (9) effectively represent just one equation as follows.

(10)

Differentiating (10) with respect to x² we get

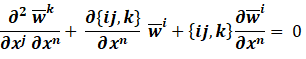(10a)

We also have

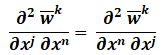(10b)

Equations (10), (10a) and (10b) lead to the following set of equations F₂.

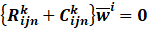(11)

where

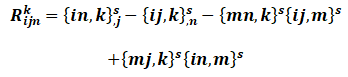(12)

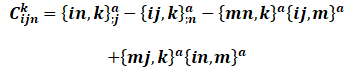(13)

Since h is the sum of a 2-form and the exterior derivative of the 1-form p, F_2, which is antisymmetric in the indices j and n, consists of (n² – n) n/2 simultaneous equations in (n² + 2n) unknowns; hence  n = 4. Therefore, F₂ determines g and h together with a velocity with which the pair of tensor fields g and h moves at a point P in 4-spacetime.

  The following equation (14)

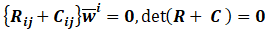(14)

Obtained by contracting F₂ with respect to k and n or k and j  determines 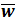at P.

According to SR, in flat spacetime symmetric metric tensor consists of 4 unit diagonal elements. The corresponding antisymmetric tensor is just the exterior derivative of the 4-element 1-form of the Maxwellean electromagnetic potentials. For these two forms of g and h, both R and C in F₂ vanish and F₂ is trivially satisfied. Accordingly, F₂ subsumes SR if the 1-form p that h contains is the set of Maxwellean electromagnetic potentials. A simple generalisation of Maxwell’s electromagnetic equations in terms of h is given in Appendix C.   

1.5 Wave aspects of both the particle and the pair of fields g and h

With the fields g and h that F₂ produces as inputs, F₁ determines an eigenvalues β and the corresponding pair of eigenvelocity vectors (𝒗, 𝒖) at P, which in ET is primarily the point of intersection of 𝒗 and 𝒖.  The field of (𝒗, 𝒖) thus produced generates a dual congruence of world lines, which is the wave aspect of the particle.

Corresponding to the above wave aspect of the particle, the velocity that F₂ produces is the velocity with which the wave aspect of the pair of tensor fields g and h moves at P.

2. Stage 2 of the Eigen Theory

2.1 The particle

The pair of vectors v and u, which represents the wave aspect of the particle, transforms into a pair of vectors v and u which represents the particle itself, as follows.

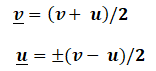(15) (16)

The set of equations F₁ in turn transforms as follows.

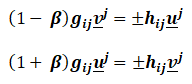(17) (18)

According to these

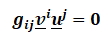(19)

Combining (17), (18) with (4) in §1.2 we get

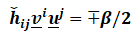(20)

Finally (1) in §1.2 becomes

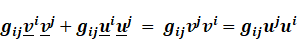(21)

In the above system of equations both + β and - βare present on an equal footing.

If the set of mutual transport equations (B15) and (B16) satisfied by v and u is transformed into the set of equations satisfied by v and u then this set does not produce the set of field equations F2  or an equivalent thereof. Therefore v and u, unlike 𝒗 and 𝒖, do not transport each other and generate a dual congruence of world lines. The pair of vectors v and u generates only a location in spacetime, which is their point of intersection. Accordingly, the pair of vector fields v and u transforms the dual congruence of line distribution, to a point-distribution, which is the particle.  This point-distribution is the microscopic equivalent of the macroscopic point-like atomic-distributions such as solid bodies.

According to (19), v and u are orthogonal with respect to the metric tensor. Therefore  and  are of translational and rotational character.

The above particle structure consists of two alternative particles that corresponds to the + and – signs of the antisymmetric tensor h. Thus, the particle structure has the potential to form a pair of ‘opposite’ particles, each in its own spacetime manifold. These two particles have - and + charges of equal magnitudes, and the mass of the first particle negligibly smaller than that of the other. The fundamental form of this pairing is the lepton-hadron pair, or the Hydrogen atom. Because in ET pairing is a fundamental feature of the physical world, owing to this fundamental pair formation by the two particles, a single stable particle does not exist in ET.

2.2 The particle centre

According to equations (17) and (18) if v and u unify then β= 0. This unified velocity, say W, is unique among the pairs of vectors v and u . Hence, its location is special among the distribution of points that makes up the particle. This special point is the particle centre, say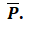 In terms of W, (17) and (18) reduce to the  following set of equations F_3.

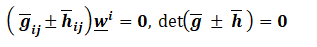(22)

Tensors 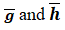in (22) are those present at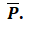. According to (22) W, represents a pair of null photon velocity vectors and in addition they are the velocities with which the pair of tensors 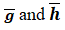moves at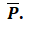Just as , associated with the wave aspect of the particle, is the velocity of the wave aspect of g and h w, associated with the particle, is the velocity of the particle aspect of g and h which behaves as a singular general tensor at the particle centre.  Notice that whilst is a distribution spread throughout the wave aspect of the particle, w is confined to the particle centre. The two values of w correspond to a photon exchange between the pair of particles mentioned in §2.1.

Equation (22) is likely to be the fundamental form of the Planck-Einstein relation.  A simple insight into this possibility based on a 2-dimensional form of (22) is given in Appendix D.

According to the foregoing, the following unifications take place at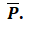

  (a)  Time and space, as w is null

  (b) Translational and rotational components of the particle motion, as v and u unify

  (c) Gravitation and electromagnetism, due to the zero determinant in (22), 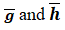acts as a singular general tensor.

2.3 Integration of the particle centre with the particle manifold

Consider the following set of equations F₂ in §1.4

(11)

If it is contracted with respect to k and n or k and j we get

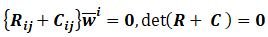(14)

With 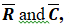which correspond to 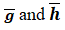at the particle centre, equation (14) becomes

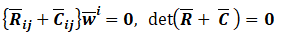(23)

At the particle centrewe also have

(22)

Since the particle centreand the null vector w present at are unique it follows that

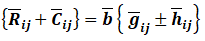(24)

where 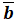is a scalar. The particle centre, subject to this set of conditions, behaves as a fulcrum on which the particle, or each of the following three pairs of opposite fields that makes up the particle, balances as a whole.   

  (a) Time and space

  (b) Translational and rotational components of the particle motion

  (c) Gravitation and electromagnetism

  In equations (24), which may be referred to as the particle-centre equation, the position of and the elements of 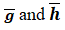at are still unknown as the base manifold in which moves is yet to be formulated. This formulation begins with the following

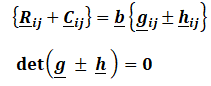(24m) (24n)

which is a generalised form of the particle-centre equation (24) in the sense that at the location of the particle centre it becomes the same as equation (24). In other words the values of 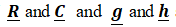at are the same as those of 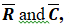and 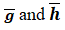. If the particle-centre equation (24) is compared to the fulcrum in a merchant’s balance then equation (24m) is comparable to the fulcrum body, which in the case of merchant’s balance can be, loosely speaking, as big as the Earth itself.

Now according to equation (24m), (g ± h) goes through a form of transformation on the left hand side of (24m) and maps to itself on the right hand side of (24m). Hence, equation (24m) is an eigen-formulation of (g ± h) with b as the corresponding eigenvalue. Just as the eigenvalue βis energy, b also is likely to be a form of energy a possible candidate for which is dark energy.

In the base manifold, which is developed in terms of equation (24m) in §3.1 below, the particle moves as a whole whilst also rotating about an axis through the particle centre.

3. Stage 3 of the Eigen Theory

3.1 The base manifold

In equation (24m), the number of elements of g and h present exceeds the number of component equations by 4. To rectify this mismatch (24m) is first split into its symmetric and antisymmetric components as follows.

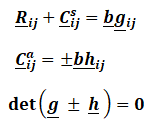(25) (26) (24n)

where

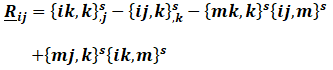(27)

(28)

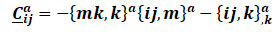(29)

(30) (31)

The 4 excess elements that equations (25) and (26) contain separate from the rest if the two components of h, the 2-form and the exterior derivative of the 1-form p, separate. With this separation (25) and (26) split into two sets of equations that represent two separate manifolds, as follows.

Set 1: h consists only of the exterior derivative of the 1-form p. Consequently C^s and C^a vanish and (25) reduces to the empty spacetime field equation in GR and (26) reduces to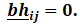Since, as outlined at the end of §1.4, the 1-form p is an integral feature of empty spacetime, b in (26) becomes zero. Then (25) and (26) reduce to just the following with respect to which the1-form p remains arbitrary.

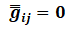(32)

As in GR, this equation produces the tensor field 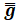characteristic of the gravitational field that the particle produces in its surrounding empty spacetime.

Set 2: Equations (25) and (26) are unchanged except that h consists only of the 2-form. Let these equations be numbered differently to avoid future confusion as follows:

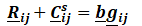(25m)

(26m)

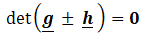(24nm)

The manifold that corresponds to this set is the base manifold of the particle. Due to the absence of the 1-form p of the Maxwellean electromagnetic potentials, base manifold is dark and its content, which is a pure field, is the ET equivalent of dark matter.

While the set 1 is the structure of the empty spacetime that envelops the particle as a whole externally, the set 2 is that of the very opposite as the base manifold envelops only the particle centre .. Therefore, the sets 1 and 2 are the outermost and the innermost structures of the particle, respectively which exists in addition to its own structure. Henceforth the empty spacetime manifold and the base manifold of the particle will be referred to as the outermost and the innermost manifolds of the particle manifold, respectively.

3.2 The complete particle structure

The three sets of equations F₂, {(25m), (26m), (24nm)} and (32) are the field equations of the particle manifold and its innermost and outermost manifolds, respectively. Now recall that in §2.2 the characteristic feature of the particle centre . is the unification thereat of each of the following three pairs of opposites.

(a) Time and space

(b) Translational and rotational components of the particle motion

(c) Gravitation and electromagnetism   

Because of these unifications, the particle-centre equation (24) is unique. The simultaneous solution of the three sets of equations (24), F₂ and {(25m), (26m), (24nm)} is the complete particle structure which includes the particle-centre position in the base manifold and the corresponding 1–form thereat. In this case (32) is redundant. While the particle centre position is characteristic of the base manifold, the 1-form thereat is characteristic of the particle manifold. Therefore the particle-centre equation (24) integrates ‘field’ and ‘particle’.  These position and 1-form are the natural counterparts of those of the point-particle in standard physics.

Notice that in ET, a single particle, owing to the absence of a second particle, cannot produce exchange-photons. As these exchange-photons are primarily associated with rotations this single particle is spherically symmetric and static at its simplest. Its centre is located at the origin of the system of coordinates.

3.3 The spherically symmetric cosmic central body system

If the particle is spherically symmetric then the particle centre is the same as the origin of the spherical polar coordinate system (t, r, θ, ∅ ).  If this spherically symmetric particle is the central body of a cosmic central body system, then a satellite would be a point particle of negligible mass in comparison to the mass of the central body.

The point-satellite moves in the innermost and the outermost manifolds of the central body, outlined in §3.1. In accord with the spherical symmetry of the central body, if the satellite moves in a circle, then simply both the innermost and the outermost manifolds contribute to it (§5.3). In this context, notice that virtually all satellites in practice move in approximate circular paths around the central body. However, in general, the motion of the satellite would not be strictly circular and as a result, in the case of the simplest form of the central body system mentioned above, the satellite motion would be constrained as follows.

(a) When the satellite is in close proximity to the central body, it effectively moves only in the outermost manifold.  An example is the motion of a satellite in the solar system (§5.3)

(b) When the satellite is sufficiently far away from the central body, it effectively moves only in the innermost manifold. An example is a satellite in a galactic system, which is sufficiently far away from the galactic centre (§5.3).

The occurrence of these constraints is due to the reduction of the satellite to a point. Otherwise the combined solution of the six sets of equations, consisting of the pair of particle-centre equations (24), pair of particle manifold field equations F_2, and the pair of field equations of the innermost and the outermost manifolds that the two particles share determines the motions of both particles, the central body and the satellite. In this case, each of these two bodies, which in reality is a collection of vast number of atoms, is approximately represented in terms of the particle in ET.

A possibility exists that, just as in GR, the pair of particles may not have an analytic solution of their own.

4. Simple applications of ET

4.1 The principle of uncertainty

In §2.1 it became clear that a stable single particle does not exist. Accordingly, the quantum mechanical uncertainty of position and momentum of a particle is primarily due to the fundamentally non-existent state of a single particle of matter, in the form of a point, occupying the centre stage of standard physics.

4.2 Matter-antimatter

Whilst the pair of equations (A14) and (A15) represents the wave aspect of the particle, the following pair of equations represents the particle.

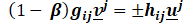(17)

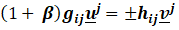(18)

According to equations (17) and (18), corresponding to the + or – signs of h, the particle consists of two alternative components rotating in opposite directions and possessing the same mass but opposite charges. These are the properties of a pair of conventional matter and antimatter particles. Therefore, in ET, a pair of matter and antimatter particles has its origin as two alternatives that are as the two sides of the proverbial coin.

These alternatives, each in its own spacetime manifold, can co-exist in a suitable base manifold producing a pair of matter and antimatter particles.

4.3 The double-slit experiment

4.3.1 Particles of matter

As mentioned in §4.1 a single stable particle does not exist. However, if circumstances such as those associated with the double-slit experiment force a particle to separate from other particles, it would only exist as its wave aspect, which is a pair of velocity vectors that transport each other. It is invisible, as unlike the particle it does not possess photon velocity vectors. Being a wave, it would go through both slits in the double-slit experiment. However, unlike the particle, the wave aspect of the particle is inherently unstable, as it has no fulcrum on which its pair of velocity vectors can balance. Hence, if observed this wave aspect converts to the particle as observation entails photons that only the particle can emit. Then along with the wave aspect the associated interference pattern disappears.   

4.3.2 Photons

Because of the integration of the wave and particle aspects of the pair of fields g and h in §2.3, the particle motion (or the photon motion) of this field at the particle centre changes over to a wave-motion elsewhere, which includes both the particle manifold and the outermost manifold which is empty spacetime. This behaviour accords with Planck’s view on radiation-behaviour.

Since for the photon velocity vector each of the three fundamental pairs of opposites are in a state of unification (§2.2), bizarre double-slit experimental may result under unusual experimental setups.  

4.4 ET and Newtonian Mechanics

ET has three fundamental sets of equations F₁, F₂, and F_3. A one-to-one correspondence exists between these and the 3 laws of motion of Newton, as follows.

F1 determines the primary motion as a pair of translational velocities	First law states that the primary motion is a single uniform translational velocity
F2 determines the force field that determines the primary motion.	Second law states the force that alters the primary motion
F3 determines a pair of photons oppositely directed in space.	Third law states that forces occur in pairs that are equal and opposite.

The above comparison between ET and Newton’s physics indicates that theoretical physics may now have journeyed a full circle and stands liberated high above the place on which she stood restricted at first.

5 Application of ET to a cosmic central body system

5.1 Planetary and galactic systems

The simplest cosmic central body system is the one in which the central body and its innermost and outermost manifolds are static and spherically symmetric and the satellites are point-particles of masses negligible in comparison to the mass of the central body. In this case, the central body and its innermost manifold, share a common centre, which is at spatial rest.

Now because of the spherical symmetry the Newtonian forces of gravity cancel at the common centre. Therefore, at this centre spacetime is well behaved from the Newtonian viewpoint, which however is contrary to what the Schwarzschild metric of GR predicts. Nonetheless, fundamentally this metric is valid only in the outermost manifold which surrounds a gravitating body and extending its validity to the interior of the gravitating body is no more than an act of pure assumption. In §5.2 below spacetime is indeed found to be well behaved at the above-mentioned common centre. In addition, the metrics of the central body and its innermost manifold may have the same form under static spherically symmetric conditions and this form is the exact opposite of the Schwarzschild metric characteristic of the outermost manifold of the central body.

5.2 The field solutions of the three manifolds

5.2.1 Field solution of the outermost manifold of the central body

The solution of (32) in terms of spherical polar coordinates (t, r, θ, ∅ ) is the following Schwarzschild metric. The parameter m is the length equivalent of the central body mass.

(33)

5.2.2 Field solution of the innermost manifold of the central body

Appendix E produces the following solution of (25m), (26m) and (24nm) in terms of spherical polar coordinates (t, r, θ, ∅ ). The eigenvalue  is zero.

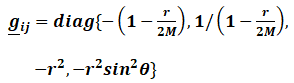(E20)

(E21)

The parameter M is the length equivalent of the ‘dark matter’ mass. Only the non-zero elements of h are shown in (E21). At the manifold centre 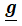is (-1,1, 0,0) and in h only the two elements are non-zero.

5.2.3 Field solution of the central body manifold This field, in general, is given by the following set of equations F₂.

(11)

However in this case the fields g and h of the central body manifold appear to be the same as (E20) and (E21) with M replaced by m, for the following reasons.

According to (E20), the innermost manifold has an event horizon of radius double the ‘dark matter’ mass. According to (33) the outermost manifold that envelops the central body also has an event horizon of radius double the central body mass. However, (33) can also represent the outermost manifold that envelops the innermost manifold in which case m becomes replaced by M. Therefore, each of these two event horizons, in effect, refers to the interface between two manifolds one of which is empty and surrounds the other, which is a dark matter manifold.

From the foregoing it may be inferred that the central body manifold for the condition of static spherical symmetry is the same as the dark matter manifold with M replaced by m. Accordingly, spacetime is well-behaved at the centre of spherical symmetry of the central body

Finally, according to (E20) the spacetime curvature, or the gravity force, increases with increasing radial distance, which is also the way the strong force acts. Therefore, it is reasonable to surmise that strong force is of gravitational origin, and that it is the fundamental force that operates at the heart of matter.

5.3 Circular motion of a satellite of mass, negligible in comparison to the central body mass

Let v and u be the velocities of the satellite in the outermost manifold and the innermost manifold, respectively.  In terms of spherical polar coordinates (t, r, θ, ϕ), these have the components (v⁰, 0, 0, v³) and (u⁰, 0, 0, u³), and they satisfy the following geodesic equations.

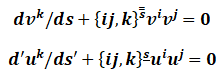(34) (35)

Symbols and 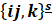are the respective connection coefficients that correspond to the two metrics (33) and (E20) in §5.2.1 and §5.2.2. Parameters s and s  in equations (34) and (35) are the respective proper times. Since the motions are circular in both manifolds, only k = 1 in (34)) and (35) needs be considered and then these equations reduce to

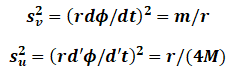(36) (37)

where r is radial distance of the satellite, and s_v and s_u are the rotational speeds that correspond to the velocities v and u respectively.

Since the satellite motion is circular, s_v in (36) is the same as that given by the Newton’s law of gravitation and m/r in (36) is the Newtonian gravitational potential at the radius r. If the density of the mass distribution in the innermost manifold is 1/( 8πMr) then s_u in (37) is also the same as that given by the Newton’s law of gravitation and r/(4M) is the Newtonian gravitational potential at the radius r.  Accordingly, two Newtonian forces act radially inwards on the satellite and the magnitude of the speed s that corresponds to the resultant of these two forces is given by

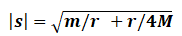(38)

According to (38), the contribution from the innermost manifold is negligible if

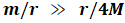(39)

This in turn means

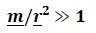(40)

where m is the normalised mass of the central body and  r  is the normalised radial distance of the satellite, normalisations being with respect to mass M and the radius 2M respectively. For any reasonable estimate of M, the value of m/r²  at the radial distance of the planet Pluto in the solar system is of the order of 10⁵. Therefore, the innermost manifold makes no appreciable contribution to the dynamics of the solar system.

5.4 Use of equation (37) to obtain the contribution made by the innermost manifold to the rotational speeds of stars in the M33 galaxy

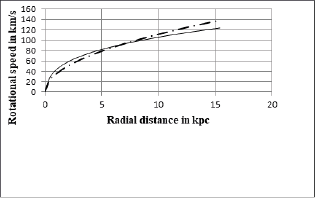
Figure 2.  The M33 galaxy

The value of M used for the computation of speeds shown in Fig.2 was obtained using (37) at the point, 6.833 kpc and 92.35 km/s of M33 galaxy data (ref.3). This value of M, which is the total dark matter mass in the physical world, is 7.47 x 10⁵⁶ g.

In Fig. 2 speeds due to dark matter obtained from observational data on the M33 galaxy are shown as a continuous curve. Speeds that (37) produces are shown as a dashed curve.    

5.5. Gravitational lensing

According to (38), for circular motion, the total gravitational potential 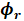at a radius r, due to both the innermost manifold and the outermost manifold, is given by

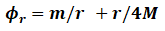(41)

Accordingly, the angle of circular deviation of a ray of light due to both manifolds is given by

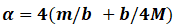(42)

where b is the impact parameter.

6. Application of ET to an Atomic Central Body System

In §5 ET was applied to a static, spherically symmetric cosmic central body system.  Here an atomic central body system is examined using the Hydrogen atom as a composite structure that represents static spherically symmetric fields. Since gravitation and electromagnetism occur inseparably, proton and electron each possess these fields. However, in a limiting form of the Hydrogen atom, proton would be purely gravitational, and electron, purely electromagnetic. Only this limiting form of the Hydrogen atom is considered here.

Following is the complete list of the fundamental particle equations.

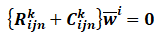(11)

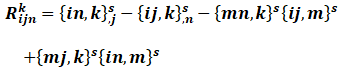(12)

(13)

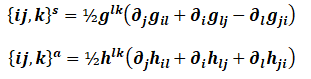(B4) (B9)

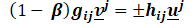(17)

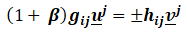(18)

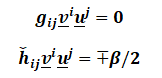(19) (20)

At the particle centre, the following equations hold.

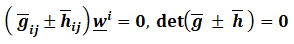(22)

  Eliminating   between (17) and (18) we get

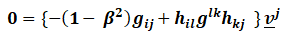(43)

For spherical symmetry, the symmetric and antisymmetric tensors g and h, in terms of spherical polar coordinates (t, r, θ, ∅),  are as follows.

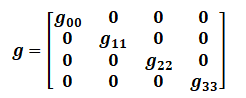(44)

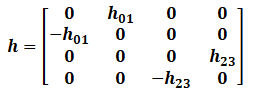(45)

Then hg^-1h within the curly brackets in (43) becomes

(46)

For the zero determinant of the tensor within the curly brackets in (43) we get

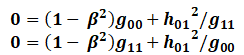(47) (48)

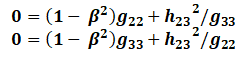(49) (50)

Due to spherical symmetry, only (47) and (48) are relevant in this case and from these we get

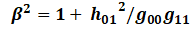(51)

The three tensor elements in (51) can be obtained by solving the field equation (11), or the following simpler equation (14), provided the conditions of g and h given below, are met.

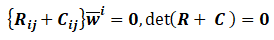(14)

(a) g and h are static and spherically symmetric

(b) g and h are approximately due to the proton and the electron, respectively.

(c) g is that present in the spacetime that envelops the proton which is located at the centre of the coordinate system. Hence, this g satisfies the ‘empty’ spacetime field equation ion GR. h is that due to the electron which occupies this ‘empty’ spacetime manifold.

These conditions are those that create a static spherically symmetric electron (manifold) of mass negligible in comparison to that of the proton present at the origin of the system of spherical polar coordinates. For these conditions equation (14) in which has only the time-component produces the following.

(a)  The Symmetric and Antisymmetric tensor fields are separate

(b)

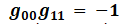(52)

(c) The coordinate r in the case of the antisymmetric tensor field becomes a constant. Since, as a result, this r cannot vary continuously it varies discretely. Hence, the electron manifold exists as a series of discrete concentric spherical surfaces.

(d) The tensor element h₀₁ becomes indeterminate

Intuitively, h₀₁ becomes indeterminate due to the unnatural static state of the electromagnetic field. Now, using findings in Quantum Mechanics the following may be assumed.

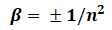(53)

where n is an integer. In this case equation (51) provides the corresponding h₀₁.

When the unity in equation (53) is replaced by α, the fine structure constant, n in equation (53) is the Hydrogen radial quantum number. When α=1 and n = 1, then |β|=1 and, as outlined in §1.2, for this condition pairing of v and u breaks down and the particle structure no longer exists.  Therefore, the least value of n is 2, which is in accordance with Balmer, Lyman and Paschen series of Hydrogen energy levels. Note that according to (53) the particle centre at which β= 0 is reached only as n tends to infinity, which is indicative of the unusual nature of the particle centre. 

7.Discussion and conclusions

The mathematical framework of ET resembles that of the fibre bundle. However, in this paper, the emphasis is on physics and not on mathematics and that was the way ET developed over a period of more than two decades.

The characteristic feature of ET is a state of dynamic balance between gravitation and electromagnetism. It leads to three progressive states of matter with a remarkably similar dynamic behaviour.

(a) The first state of matter: it consists of an alternative pair of particles that correspond to the antisymmetry of the electromagnetic field, or the + and - signs of charge.  These particles occupy spacetime manifolds of their own and this occupation is perhaps the ET equivalent of the Pauli Exclusion Principle in quantum mechanics. Both particles are made up of the following three pair of complementary opposites. 

time - space

translation - rotation

gravitation - electromagnetism

Translation and rotation unify at a special point, which is the particle centre. This state of unification results in a pair of null velocity vectors, one for each particle. Each of the remaining two pairs of opposites also unifies at the particle centre via this pair of null vectors.  Due to these three unifications, the particle centre, which acts as a fulcrum on which each pair of opposites maintains a state of dynamic balance, is of an extraordinary character.

Each particle in addition to its own spacetime manifold is endowed with two other spacetime manifolds; one, which envelops the particle as a whole, and the other, which envelops only the particle centre. They are the outermost and the innermost manifolds of the particle.  The contents of the innermost manifold are the ET equivalents of dark matter in standard physics.   

(b) The second state: the two particles of the first state are the ET progenitors of the hadron and lepton of particle physics, respectively. They do not occur singly but as a pair which is the Hydrogen atom. They share the outermost and innermost manifolds, mentioned above. In the latter, they exchange the null photons, mentioned above, and maintain a state of dynamic balance.    

(c) The third state: a macroscopic body such as a planet or the central body of a central body system    

In standard physics, rotational and translational motions are separate and so are gravitation and electromagnetism. As mentioned in the Introduction to this paper, the dynamic interaction between members of each of these two separations (along with those between time and space) is what characterises the central region of the physical world. Therefore, the inescapable conclusion is that standard physics applies only in this region whilst ET applies throughout the physical world.

In ET strong force is of gravitational origin and since weak force is already linked to the electromagnetic force, in ET only a pair of balanced forces exist, which are gravitational and electromagnetic, respectively

According to Appendix F the spacetime curvature of the global ‘dark matter’ manifold has only a marginal effect on the observed Hubble expansion of the physical universe.

According to ET, an intrinsic physical reality may exist, which in itself is inaccessible to us. It consists of a wave-particle duality of which the wave aspect consists of a field of a pair of eigenvelocity vectors v and u that exists in a state perpetual nothingness and satisfies a set of field equations F_1. The particle aspect of this duality consists of a pair of translational and rotational velocity vectors v and u and it has a unique centre characterised by a null photon velocity vector which satisfies a set of equations F_3.  A pair of symmetric and antisymmetric tensor fields that characterises F₁ and F₃ may appear at first as arbitrary_, and yet they may be simply autonomous by nature. The arbitrary perturbation of the motion of the pair of eigenvelocity vectors in §1.4 that nonetheless maintains their state of nothingness opens external access to the pair of symmetric and antisymmetric tensor fields in terms of a set of field equations F₂. This access is the cause of the extrinsic physical reality, which lends itself to observation and experimentation.  

Finally, consider the two fundamental branches of mathematics, algebra and geometry.

(a) Algebra: The primary element of the complete form of algebra is the complex number, which consists of a pair of any two numbers. A simple manipulation of the imaginary operator i, which integrates this pair of numbers establishes that, on its own, it stands for the unification of the pair of opposites +1 and -1.

(b) Geometry: The primary elements of simple geometry may be considered as a diameter and its associated circle. The length of the diameter and the circumference of the circle become unified in the transcendental number π.

The above unifiers i and π themselves become unified in the following null fashion which is a generalisation of the Euler identity. In (54) the integer n is greater than 1.

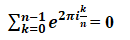(54)

From the foregoing it follows that pairing and an associated underlying unification of these pairs, is a feature shared by both mathematics and physics. The physical counterpart of the null equation (54) is the pair of null photon velocity vectors, at the particle centre, that unifies the two members of each of the three fundamental pairs of opposites, mentioned above.  

8. Future Work

The complete structure of the Hydrogen atom.

9. Acknowledgements

  I thank Dr. Edvige Corbelli [3] for promptly emailing to me the set of M33 data that I used for obtaining the curves in Fig .2.

  Without the generous encouragement and support of my wife Lalitha, this paper, which took more than two decades to complete, would not have been a possibility.

Appendix Download

References

1. George Arfken (1985) Geometrical Methods for Physicists. Academic Press Inc 235.
2. Eddington AS (1975) The Mathematical Theory of Relativity. Chelsea Publishing Company 84-85.    
3. Corbelli E., Salucci P (2000) The Extended Rotation Curve and the Dark Matter Halo of M33. Monthly Notices of the Royal Astronomical Society 311: 441- 447
4. Schutz BF (1985) A First Course in General Relativity. Cambridge University Press 255.